Evaluations for input

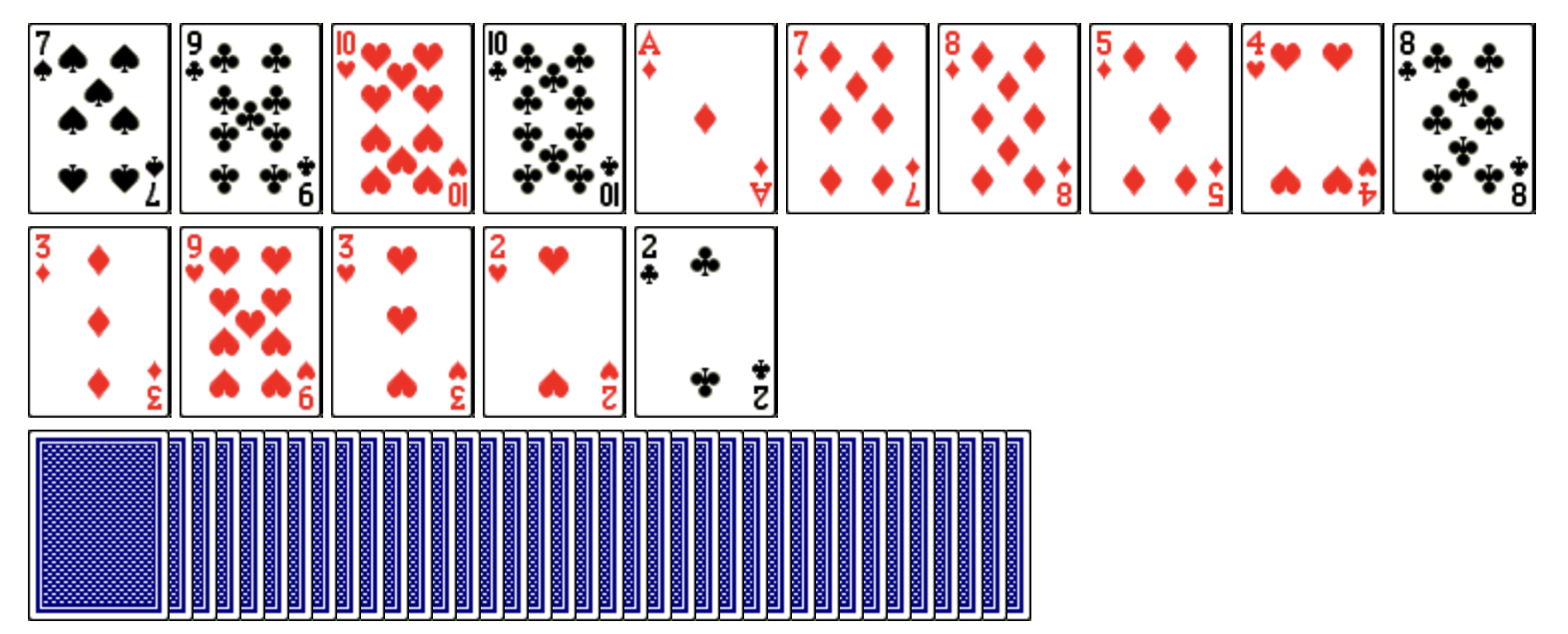The image shows a collection of playing cards from a standard 52-card deck. At the top, there are 16 cards displayed, consisting of hearts, diamonds, spades, and clubs ranging in values from 2 to Ace. Below, there is a long row of around 32 face-down cards, with only the blue or violet backs visible.

In total, there appear to be approximately 48 cards shown in the image, which is very close to a full 52-card deck used for many classic card games.

The image shows a set of playing cards arranged in two rows. The top row contains 10 cards, from left to right:

7 of Spades 9 of Clubs 10 of Hearts 10 of Diamonds Ace of Diamonds 7 of Hearts 8 of Diamonds 5 of Hearts 4 of Spades 8 of Hearts

The bottom row has 5 cards:

3 of Diamonds 9 of Hearts 3 of Hearts 2 of Hearts 2 of Diamonds

Assuming Aces are worth 1, and face cards (Jack, Queen, King) are worth 10, the total count of the displayed cards is:

7 + 9 + 10 + 10 + 1 + 7 + 8 + 5 + 4 + 8 + 3 + 9 + 3 + 2 + 2 = 88

Therefore, the total count of all the cards shown is 88.

The image shows playing cards spread out in two rows, the top row with face-up cards and the bottom row with face-down cards showing the blue back design.

From left to right, the face-up cards are:

• 7 of Spades
• 9 of Clubs
• 10 of Hearts
• 10 of Clubs
• Ace of Diamonds
• 7 of Diamonds
• 8 of Diamonds
• 5 of Diamonds
• 4 of Hearts
• 8 of Clubs

In the second row, from left to right, the face-up cards are:

• 3 of Diamonds
• 9 of Hearts
• 3 of Hearts
• 2 of Hearts
• 2 of Clubs

The face-down cards cannot be identified as their faces are hidden.

The total count of the face-up cards is calculated by adding the values of each card:

• For numbered cards, the value is their face number.
• For Aces, the value is typically either 1 or 11 depending on the game, but we'll use the most common value of 1.
• For face cards (J, Q, K), which are not present here, the value is usually 10.

Let's add up the face-up card values: 7 (Spades) + 9 (Clubs) + 10 (Hearts) + 10 (Clubs) + 1 (Ace of Diamonds) + 7 (Diamonds) + 8 (Diamonds) + 5 (Diamonds) + 4 (Hearts) + 8 (Clubs) + 3 (Diamonds) + 9 (Hearts) + 3 (Hearts) + 2 (Hearts) + 2 (Clubs)

This equals: 7 + 9 + 10 + 10 + 1 + 7 + 8 + 5 + 4 + 8 + 3 + 9 + 3 + 2 + 2 = 88

The total count for the face-up cards is 88.